The source space in individual FEM head models
| Module information | |
| Modules | BESA Research Standard or higher |
| Version | BESA Research 6.1 or higher |
Leadfield table and source space definition
In the FEM leadfield generation workstep of BESA MRI, a leadfield table (.lft) and a description of sources (.loc) can be generated. The leadfield table contains the simulated EEG potentials or MEG signals for sources in x-, y-, and z-direction distributed on a regular grid covering the entire source space. The source space definition file specifies the locations of the sources and information on the neighbor nodes for each grid node.
In the source analysis module of BESA Research, the information of leadfield table and the source space definition is loaded for individual FEM head models. During dipole fitting BESA Research computes the EEG potentials or MEG signals for any given dipole source employing cubic Bezier-spline interpolation. This way, the individual FEM model can be used for the source analysis like any of the other head models.
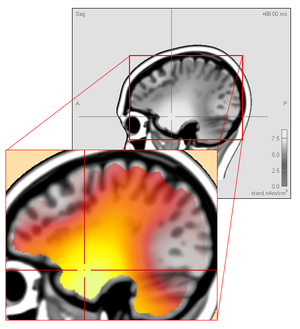
Why is the source space of FEM head models slightly smaller than the brain area in MRI?
When a FEM head model is used in the source analysis module of BESA Research, the source space are slightly smaller than the brain area in MRI (see the example screenshot below).
This is because in FEM source modeling the location of the sources are placed inside of the brain and sufficiently apart from non-brain compartments (CSF and skull). In other words, the finite element nodes close to the sources should belong to the brain. This condition has to be fulfilled to avoid unrealistic source modeling and numerical problems for the Venant dipole modeling approach (Lew et al., 2009; Vorwerk 2016).
See also
References
- Lew, S., Wolters, C., Dierkes, T., Röer, C., MacLeod, R. (2009). Accuracy and run-time comparison for different potential approaches and iterative solvers in finite element method based EEG source analysis. Appl. Numer. Math. 59 (8), 1970–1988.
- Vorwerk, J. (2016). New Finite Element Methods to Solve the EEG/MEG Forward Problem. Ph.D. thesis in Mathematics, Westfälische Wilhelms-Universität Münster.